Engine (work & efficiency)
![]() Near field: For simplicity, let us consider a material with a single dominant frequency
Near field: For simplicity, let us consider a material with a single dominant frequency ![]() ,
,
where the propulsive force and heat transfer in the near-field regime can be estimated as
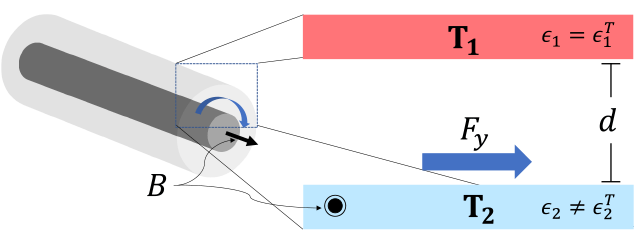
 ,
, ![]()
where C is dimensionless, andis a measure of asymmetry, e.g..
in a magnetic field.
![]() Work can be extracted from the motive force, only if the plate is moves with some velocity v
Work can be extracted from the motive force, only if the plate is moves with some velocity v
Naively, from the extracted power, the engine efficiency is given by
The above formula may suggest exceeding the Carnot efficiency for velocities greater than
![]() The Rytov formalism combined with Linear response relations, provides the needed corrections:
The Rytov formalism combined with Linear response relations, provides the needed corrections:
Heat emitted by a moving body is larger than when at rest:
There are frictional forces (even with a vacuum gap) reducing the propulsive force to
, with friction coefficient
Together, these correct the expression for the efficiency of
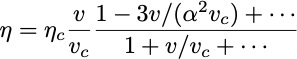
For maximum power
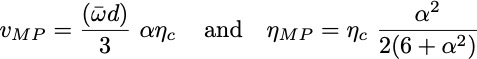
Fluctuations and linear Response
![]() Rytov formalism allows computation of fluctuations in force and heat, both in and out of thermal equilibrium
Rytov formalism allows computation of fluctuations in force and heat, both in and out of thermal equilibrium
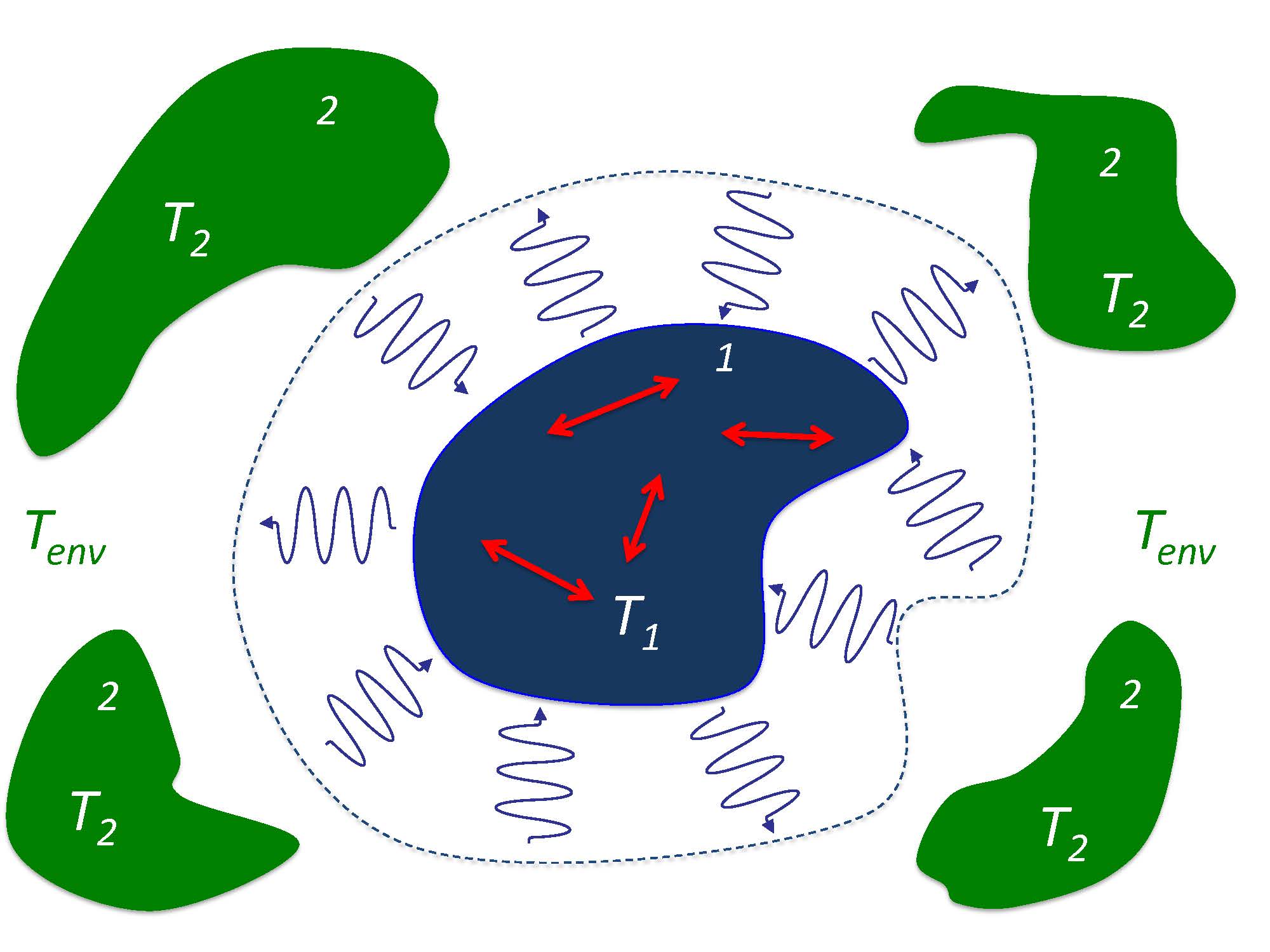
_.jpeg)
![]() Linear response (Onsager relations) connect equilibrium fluctuations to responses to small perturbations:
Linear response (Onsager relations) connect equilibrium fluctuations to responses to small perturbations:
Friction coefficient in vacuum at finite temperature
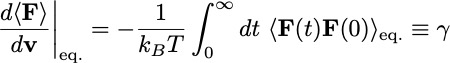
Green Kubo relation (explicitly confirmed) provide coefficient of thermal conductivity
Onsager relation (relying of symmetry of mixed expectation values) give
"Nonequilibrium Fluctuational QED: Heat Radiation, Heat Transfer and Force,"
V. Golyk, M. Krüger, and M. Kardar, Phys. Rev. B 88, 155117 (2013). (offline)